A well-centered polytope is one whose vertices all lie on a sphere (also known as being circumscribable, or equiradial) and with the center of that sphere (the circumcenter) within the polytope’s interior.

Circumscribable polygons are also known as cyclic polygons.

There are circumscribable polytopes which are not well-centered, like this isosceles trapezoid:

Or the equilateral pentagonal pyramid (Johnson solid J2):

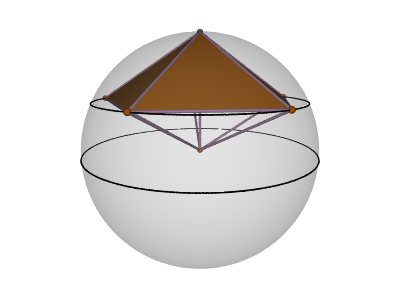
A polytope is vertex-transitive if the polytope can be rotated or reflected to take any vertex to any other vertex, while the whole polytope looks the same as when you started; then we say “the polytope’s symmetries act transitively on its vertices”. This is also known as being isogonal.

A vertex-transitive polytope is always well-centered. But there are also well-centered polytopes which aren’t vertex-transitive, like this pentagon:

An \(n\)-gonal bipyramid can also be made well-centered for any \(n\), by putting the apices on the sphere centered on the base polygon’s circumcenter and going through the base’s circumcircle. These won’t be vertex-transitive, though, since the apices are different from the vertices on the base—except for the square bipyramid; when you scale that to be circumscribable, you get a regular octahedron.

The faces of a well-centered polytope aren’t necessarily well-centered themselves, although they are circumscribable. For instance, you can make a well-centered hexahedron with four sides being the isosceles trapezoid we saw above. Just attach four of them in a cycle, alternating long and short edges; the top and bottom will form rectangles, oriented perpendicular to eachother:

(This shape could be called a disphenoid frustum, if you’re so inclined. It’s also a prismoid.)

A well-centered polytope all of whose faces are themselves well-centered is called completely well-centered.

So, a vertex-transitive polytope is always well-centered. And its faces are always circumscribable. But its faces aren’t necessarily well-centered; for instance, the disphenoid frustum we just saw is vertex-transitive, but the trapezoid sides aren’t well-centered.

So vertex-transitive does not imply completely well-centered. Also, being completely well-centered does not imply vertex transitivity; the pseudorhombicuboctahedron is one completely well-centered but not isogonal polytope. Johnson solids J11 (Gyroelongated pentagonal pyramid) and J19 (Elongated square cupola) are more examples.

Does adding some more conditions allow one to draw conclusions in one direction or the other?

For instance, a circumscribable and equilateral polytope must actually have regular 2-faces (since equilateral cyclic polygons are regular). So the 3-faces must be regular-faced and circumscribable, which means they can only be among the Platonic solids, the Archimedean solids, 25 out of the 92 Johnson solids, an \(n\)-gonal uniform prism, or an \(n\)-gonal equilateral antiprism.

Isogonal + Isotoxal ⇒ completely well-centered?

Could it be that a polytope which is both vertex- and edge-transitive is completely well-centered? Such a polytope is circumscribable and equilateral, so it has regular 2-faces. If the 3-faces must be well-centered then that would rule out several of the 25 circumscribable Johnson solids.

A counterexample would have to be at least 4-dimensional. A 4-polytope which is vertex- and edge-transitive but not completely well-centered would have to have non-well-centered regular-faced polyhedra for some of its 3-faces. There are actually only three possible polyhedra: J1, the square pyramid; J2, the pentagonal pyramid; or J6, the pentagonal rotunda.

We saw in Twisty Antiprisms that there’s no vertex-transitive 4-polytope made only of J2. But we haven’t ruled out a mix of J2 and other facets.

Well-centered j-faces ⇒ well-centered (j+1)-faces?

Could it be true that a vertex-transitive polytope with well-centered 2-faces always has well-centered 3-faces? (Or, more generally, that a vertex-transitive polytope with well-centered \(j\)-faces (\(j \geq 2\)) always has well-centered \((j+1)\)-faces?)

A potential counterexample to this last conjecture is a 4-polytope which has been called spidrox, or the “Swirlprismatodiminished Rectified 600-cell”. This is reported to be vertex-transitive and to have equilateral triangles, squares, and regular pentagons as 2-faces, so its 2-faces are all well-centered. Yet its 3-faces include square pyramids, which are not well-centered. In a future installment we’ll investigate this polytope with polymake.